Deconvolution

The input impulse response of a system is defined as the reflections resulting from excitation by an ideal acoustic impulse. The acoustic pulse that is produced experimentally is not an ideal impulse because of its finite duration. To get the input impulse response, we need to deconvolve the pulse entering the object's input from the reflections which return to the object's input. However, the measurement we make is of the object reflections when they have experienced losses corresponding to travel down the distance $l_2$ back to the microphone. By terminating the source tube in a flat plate or cap, we can give a 100$\%$ reflection of the input pulse down the same length of tube to the microphone. This measurement is referred to as the calibration pulse.

The input impulse response at the input plane is the deconvolution of the backward and forward going signals there. Our measurement records these signals once they have travelled an extra distance of $l_2$. In order to recover the signals present at the input plane we could apply the same loss filter to both. This corresponds to multiplying both by the same function in the frequency domain. Since deconvolution is frequency domain division, the effect of the loss filter will be divided out. The input impulse response is therefore equal to the deconvolution of the signals measured at the microphone:

$$IIR(\omega)= \frac{R(\omega)I^{*}(\omega)}{I(\omega)I^{*}(\omega) + q}$$ (5.29)

where $I$ is the Fourier transform of the calibration pressure pulse and $R$ is the Fourier transform of the reflected pressure signal. $q$ is a constraining factor used to prevent division by zero which would otherwise occur since the calibration pulse measurement consists only of background noise at high frequencies. In practice it low pass filters the input impulse response, removing high frequency noise. Choosing too large a value for $q$ introduces errors into the deconvolution. For the current set up, $q = 0.00001$ was found to remove much of the high frequency noise, with a small change in $q$ having no effect on the input impulse response within the bandwidth of our calibration pulse.

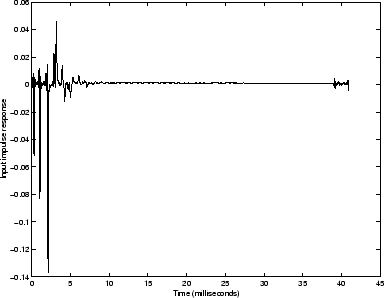
Figure 5.6 shows a measurement of the calibration pulse and figure 5.7 shows the input impulse response resulting from the deconvolution of the calibration pulse from the object reflections. The characteristic shape of the calibration pulse has been removed from the object reflections, making the individual reflections from the steps in the bore impulsive as is expected.

Figure 5.6: Calibration pulse

Figure 5.7: Input impulse response