Pressure radiation from a piston terminated in an infinite baffle

Consider a rigid piston in a rigid infinite baffle as shown in figure 3.1. The piston vibrates uniformly with a sinusoidal velocity of amplitude $v$ normal to the baffle.

Figure 3.1: Piston in an infinite baffle

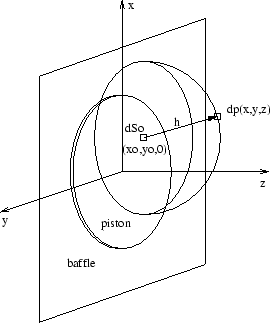In order to calculate the behaviour of this system, we split the piston into infinitesimal simple source elements and sum the resulting pressure fields. A piston surface element of area $dS_0$ is present at $(x_0,y_0,0)$. This surface element oscillates with a velocity amplitude of $v$ normal to the baffle and acts as a simple source of spherical pressure waves. These are represented on the diagram by a hemispherical shell, with the acoustic pressure at a distance $h=\sqrt{(x-x_0)^2 + (y-y_0)^2 + z^2}$ from the source element given by [40]

$$dp(x,y,z) = i \omega \rho \frac{Q}{2\pi} \frac{e^{-ikh}}{h},$$ (3.2)

where $Q=v dS_0$ is the simple source strength and a $e^{i\omega t}$ time factor is assumed throughout. The part $e^{-ikh}/h$ is known as the Green's function and implies that the pressure oscillates sinusoidally in space with wavelength $\lambda = 2 \pi/k$ and with an amplitude that dies as $1/h$. Integrating (3.2) over $S$, the surface of the whole piston, we get the total pressure field due to the sum of all the source elements that make up the piston.

$$p(x,y,z) = i \omega \rho \frac{v}{2\pi} \int_S dS_0 \frac{e^{-ikh}}{h}.$$ (3.3)

Note that the integrand is singular (tends to infinity) as $h$ tends to zero. This problem must be addressed before numerical integration is possible.